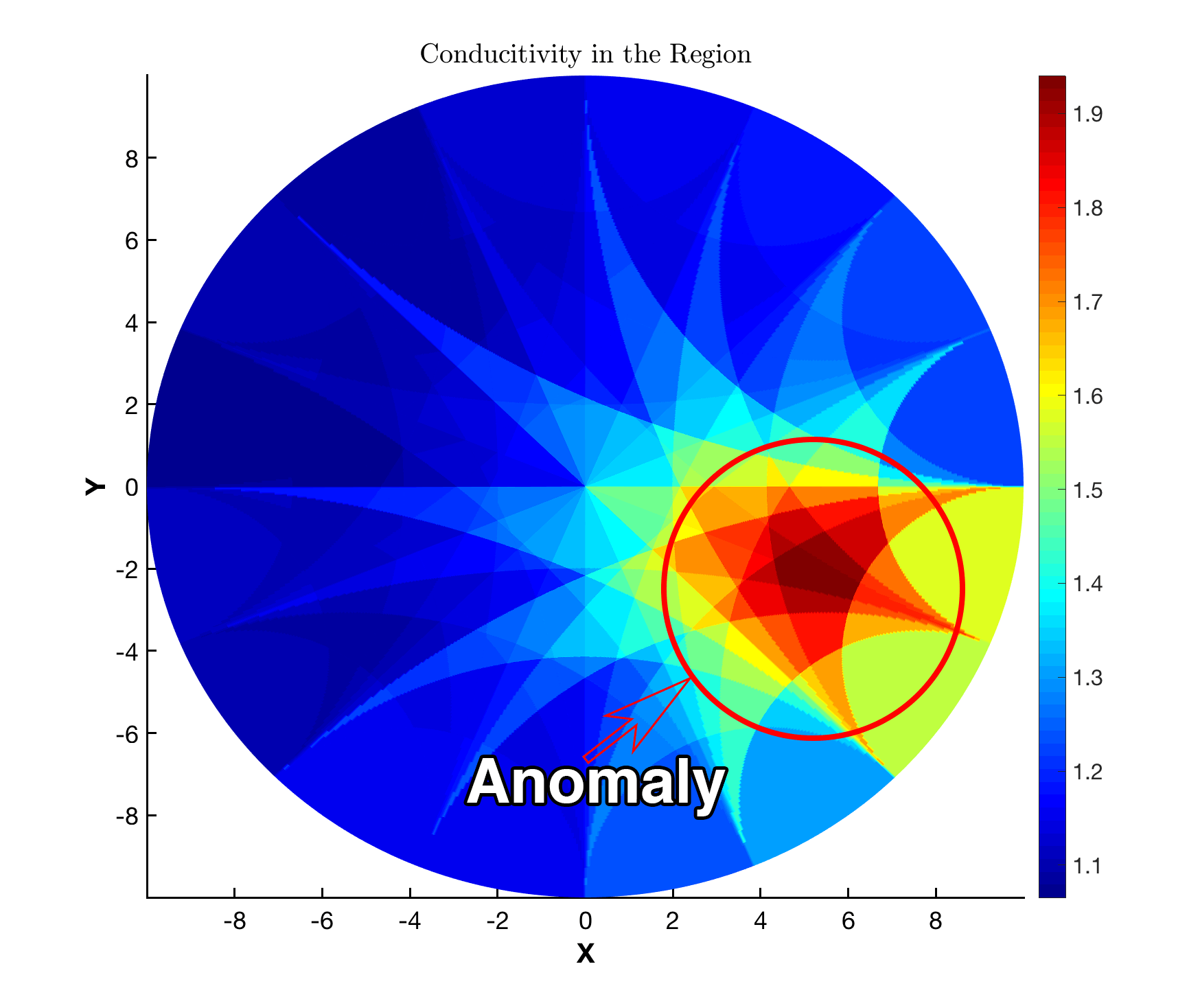
This was a project done for Math 406 at UBC. The project involved using EIT data and computational/numeric method to back propagate to an underlying anomaly
The preamble of the project: In this project we illustrate the application of Green’s functions to the problem of determining changes of conductivity σ(r, θ) within a circular region from a finite number of voltage measurement along the boundary for a current I applied to parts of the boundary. This problem is a so-called inverse problem in which material properties are determined from measurements of field quantities. This process can typically lead to images of anomalies that it is important to identify - for example, tumors are known to have significantly higher conductivities than normal tissue thus being able to identify regions with perturbed conductivities can lead to images of tumors. Other applications could be the identification of plastic (low conductivity) land- mines from the surrounding soil in which they are buried, or changes in conductivities to identify mineral deposits.